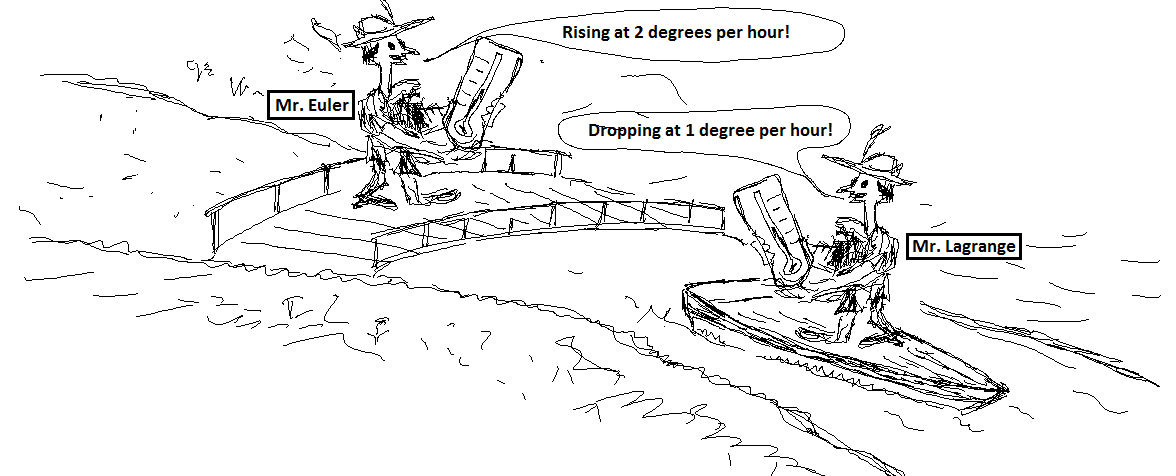
Imagine two people, Mr. Euler and Mr. Lagrange, making temperature observations in order to calculate the rate at which the temperature is varying. Mr. Euler is standing on a bridge, while Mr. Lagrange is in a boat carried by a river, as shown in the figure. When the boat passes the bridge, they discover that their observed rates differ. Why?
This happens because the temperature differs in different locations on the ground. To calculate the rate, they divide the difference between the two observed temperatures by the time interval between the measurements. Even if one of the measurements was made when the boat was passing the bridge, the other measurement was when the boat was away from the bridge. Then, even if they took this other measurements at the same instant, the results can differ.
In the example with the boat it was assumed that the boat was carried by the fluid, that is, it was moving with the velocity of the fluid itself. The Eulerian viewpoint consists in considering quantities as dependent on time and point in space. The Lagrangian viewpoint consists in considering quantities as dependent on time and the fluid particle. Lagrangian viewpoint is particularly useful when the laws of fluid motion are derived from the Newton laws. Once derived, however, these laws are more convenient to use from the Eulerian viewpoint.
We will now introduce a simple notation, which will be convenient in what follows.
In general, the temperature T of the fluid varies from point to point and is therefore a field: T = T(t,x). The rate of change is a derivative; in this case it is the derivative with respect to time. As we just discussed, at the same point x we can have two different values of time derivative, the one obtained from the Eulerian viewpoint and the other obtained from the Lagrangian viewpoint. The Lagrangian derivative is denoted in the standard way: for example, the time derivative of temperature T is denoted dT/dt. The Eulerian, also called local, derivative is denoted similarly, but using a special form of the letter “d”: ∂T/∂t.
If ∂T/∂t = 0 then the field T is independent of time, so that we can say that T = T(x). Such fields are called steady.
If dT/dt = 0 then T at each fluid particle is independent of time. Such fields are called frozen. One can imagine that the values of the field quantities were glued by frost to fluid particles and move with them.
Finally, the difference between these two derivatives is called a convective derivative and denoted as u·∇T, where u is the velocity field of the fluid particles, and ∇ is a special symbol called nabla. This notation is introduced such that the material derivative is a sum of the local derivative and the convective derivatives:
dT/dt = ∂T/∂t + u·∇T.
Of course, the rate of change, or derivative, can be calculated for other quantities, for example, for the velocity itself, and then
du/dt = ∂u/∂t + u·∇u.
If no forces were acting on fluid particles then, according Newton’s first law of motion, each particle would move with constant speed, and the material derivative of the velocity would be zero: du/dt = 0. The local derivative, however, would not be necessarily zero, since we would have
∂u/∂t = –u·∇u.
The above equation means, therefore, that the velocity is changing at a fixed point in space only because fluid particles with different velocities are passing through this point of space, while being carried, or advected, by the fluid.
In reality, of course, there are forces acting on the fluid particles, and these forces will be discussed in the following pages.